
Year Three - Seeing Fractions: The parts and the wholes
Home - Seeing Fractions: The parts and the wholes Map
About this Big Idea
In this Big Idea students explore unit fractions other than 1⁄2 as they expand their understanding of fractions. Students will investigate the utility of unit fractions in a range of situations through visualisations and by exploring patterns.
Understanding Goals:
Students will understand:
- that a fraction is a relationship between the numerator and denominator.
- that a fraction is one number, not two.
- unit fractions from 1⁄1 to 1⁄6.
Background:
In Stage 2, fractions with denominators of 2, 3, 4, 5, 6, 8, 10 and 100 are studied. Fractions are used in different ways: to describe equal parts of a whole; to describe equal parts of a collection of objects; to denote numbers (eg 1⁄2 is midway between 0 and 1 on the number line); and as operators related to division (eg dividing a number in half).
A unit fraction is any proper fraction in which the numerator is 1, eg 1⁄2, 1⁄3, 1⁄4, 1⁄5, etc
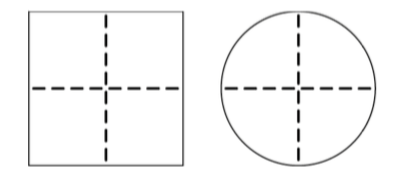
The continuous model area uses multi-directional cuts or folds to compare fractional parts to the whole. This model should be introduced once students have an understanding of the concept of area in Stage 2.
Core Content from the Syllabus:
Working Mathematically
Fractions & Decimals
- recognise that as the number of parts that a whole is divided into becomes larger, the size of each part becomes smaller (Reasoning)
- recognise that fractions are used to describe one or more parts of a whole where the parts are equal,
- interpret the denominator as the number of equal parts a whole has been divided into
- interpret the numerator as the number of equal fractional parts
- model and represent unit fractions, including 1⁄2, 1⁄3, 1⁄4, 1⁄5 and their multiples, to a complete whole
- model, compare and represent fractions with denominators of 2, 3, 4, 5, 6, 8, 10 and 100
Length
- use the term 'perimeter' to describe the total distance around a two-dimensional shape
- estimate and measure the perimeters of two-dimensional shapes
Language:
- whole, part, equal parts, half, quarter, eighth, third, sixth, fifth, tenth, hundredth, one-sixth, one-tenth, one-hundredth, fraction, numerator, denominator, unit fraction, whole number
- perimeter, length, unit,
Connected to:
Mindset Mathematics Learning Activities

Visualise
Students explore unit fractions and the relationship between part and whole by creating visual models using pattern blocks. - See page 185
Questions for reflection:
- What do the representations of 1⁄3 have in common?
- How do you know each is 1⁄3?
- What makes 1⁄3?
- What kind of shapes can you make where the blue rhombus is 1⁄3? How could you change the shape so that the blue rhombus becomes a different fraction?

Play
In the game Cover Up, students play with finding unit fractions in a complex geometric image and work together to try and cover the board. Then they cut out the shapes they made and sort them by unit fractions to explore patterns and develop strategies for playing the game. - See page 191
Questions for reflection:
- What strategies did you develop for playing the game?
- How did you see fractions?
- What fractions were easier or harder to find? Why?
- What patterns did you notice? How might these patterns help you play the game?
- If you were teaching someone how to play Cover Up, what is the one strategy you think they ought to know? Why?

Investigate
Students connect fractions to distance and the number line by finding as many different rectangles as they can with a perimeter of 17 units. - See page 202
Questions for reflection:
- What rectangles did you find? How did you now the perimeter was 17 units?
- What made finding these rectangles challenging?
- Do you think you found all the possible rectangles? Why or why not?
- If we wanted to find them all, what could we do?
- What patterns do you notice in the perimeters that require fractional side lengths?
- What other patterns do you see?
- Can we find all the rectangles for a given perimeter? Why or why not?
- How are fractions connected to length?
Credit:
Boaler, Munson & Williams (2018) - Mindset Mathematics: Visualizing and investigating big ideas Grade 5
NESA - Mathematics K-10 - 2012






