
Year Five - Using Fraction Equivalence
Home - Using Fractions Equivalence Map
About this Big Idea
In this Big Idea students explore the concept of fraction equivalence and build an understanding that a fraction represents one number defined by the relationship between its two parts (numerator & denominator).
Understanding Goals:
Students will understand:
- that a fraction represents one number, not two, and
- that the single number comes from a relationship between the numerator and the denominator.
Background:
In Stage 3 Fractions and Decimals, students study fractions with denominators of 2, 3, 4, 5, 6, 8, 10, 12 and 100. Fractions may be interpreted in different ways depending on the context.
Core Content from the Syllabus:
Working Mathematically
Fractions
- explain or demonstrate why two fractions are or are not equivalent (Communicating, Reasoning)
- find equivalent fractions by re-dividing the whole, using diagrams and number lines
- record equivalent fractions using diagrams and numerals
- develop mental strategies for generating equivalent fractions, such as multiplying or dividing the numerator and the denominator by the same number
- compare the relative size of fractions drawn on the same diagram
- investigate and explain the relationship between the value of a unit fraction and its denominator (Communicating, Reasoning)
- Solve problems involving addition and subtraction of fractions with the same or related denominators
- use knowledge of equivalence to simplify answers when adding and subtracting fractions
Area
- establish the relationship between the lengths, widths and areas of rectangles (including squares)
Visual Art:
- discusses the artist’s intention and/or the use of styles and techniques in selected works and considers the possible meanings of these works
- identifies and describes the properties of different forms, materials and techniques in artworks and comments on how these are employed in the representation of subject matter
Language:
- whole, equal parts, half, quarter, eighth, third, sixth, twelfth, fifth, tenth, hundredth, thousandth, one-thousandth, fraction, numerator, denominator, mixed numeral, whole number, proper fraction, improper fraction, equivalent, simplest form
Connected to:
Mindset Mathematics Learning Activities

Visualise
Exploring the colors used in geometric art, students build on their understanding of fraction equivalence to visualize the need for common denominators when adding and subtracting fractions. - See page 83
Questions for reflection:
- How did you approach solving these problems? What strategies did you develop?
- How did you name the fraction for each color so you could join them? How did equivalent fractions help you?
- What did you have to do in order to solve these problems?
- Why does the denominator matter when adding or subtracting fractions?

Play
Building on students' work with adding and subtracting fractions in geometric art, students create “good” and“ close” fake Mondrian paintings. The class does detective work to find the close fakes, which are more than 1/2 color. - See page 94
Questions for reflection:
- What did you have to think about when you were making your fakes— either the good one or the close one?
- How did you use fractions to help you as you planned and created your paintings?
- If you were given two fractions to add, such as 3/5 and 1/6, what would you have to think about in order to find their sum?

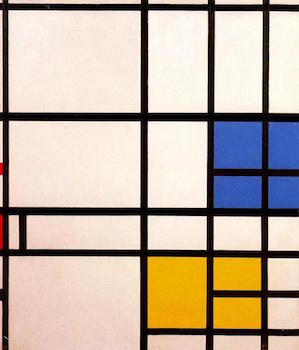

Image by Piet Mondrian - Image 1 - Broadway Boogie Wogie, Image 2 - Composition London, Image 3 - Composition No. 3

Investigate
Students bring together their understanding of fraction equivalence and fraction addition and subtraction to investigate partitioning squares and comparing the regions inside. - See page 101
Questions for reflection:
- How did you have to think about the fractions to find a difference of 1/4?
- What different ways did you find to partition squares?
- What patterns were you using to help you? What patterns did you notice?
- What size squares did we find solutions for? Which sizes did we not find solutions for? Why do you think that is?
- Where can you see equivalent fractions in this investigation? How might they help you think about the squares that do and do not work for a particular fraction difference?
Credit:
Boaler, Munson & Williams (2018) - Mindset Mathematics: Visualizing and investigating big ideas Grade 5
NESA - Mathematics K-10 - 2012








